
- Trending Categories
 Data Structure
Data Structure Networking
Networking RDBMS
RDBMS Operating System
Operating System Java
Java MS Excel
MS Excel iOS
iOS HTML
HTML CSS
CSS Android
Android Python
Python C Programming
C Programming C++
C++ C#
C# MongoDB
MongoDB MySQL
MySQL Javascript
Javascript PHP
PHPPhysics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Compound Microscope
Introduction
Compound microscope is an optical instrument used to observe microscopic objects. The scientific study of microscopic objects is called microscopy. Early microscopes had only one lens. Hence, they are now called ordinary microscopes. Compound microscopes contain at least two lenses. In 1590 Hans Janssen and his son Zacharias Janssen developed the first compound microscope in the Netherlands. Galileo Galilei between 1609 and 1624 developed the compound microscope using concave and convex lenses and studied the compound eyes of insects. In 1625, the name microscope was given by the German physician Giovanni Faber. Robert Hooke, an English microscopist, examined thin sections of cells with his compound microscope in 1665-1667.
Simple Microscope
A simple microscope is a magnifying lens with a short focal length to obtain a straight magnified optical image of an object.
Therefore, when the object is placed within the focal length of one side of the lens and viewed through the other side, the nearest point that can be clearly seen by the eye is called the near point and the farthest point is called the far point.
Resolving Power of Microscope
By looking at the object with a microscope, details about the object are obtained.
A good microscope should not only magnify the object but also resolve two points on the object that are separated by a minimum distance$\mathrm{(d_{min})}$.
Here d_min is known as resolution and its inverse is known as resolving power.
If $\mathrm{d_{min}}$ is the distance between two points on the object, the magnification m
$$\mathrm{m=\frac{r_o}{d_{min}}}$$
$$\mathrm{r_o=\frac{1.22\lambda
u}{a}}$$
$$\mathrm{d_{min}=\frac{r_o}{m}=\frac{1.22\lambda
u}{am}=\frac{1.22\lambda
u}{1.22λu}}$$
$$\mathrm{[Magnification= m=ν/u]}$$
$$\mathrm{d_{min}=\frac{1.22\lambda f}{a}\:\:\: [u \approx f]}$$
On the object side,
$$\mathrm{2\:tan \beta \approx 2\:sin\beta=\frac{a}{f}\:\:\:[a=2f\:sin \beta]}$$
$$\mathrm{d_{min}=\frac{1.22\lambda}{2\:sin\beta}}$$
To further reduce the value of the minimum distance $\mathrm{(d_{min})}$, the objective lens of the microscope should be immersed in a container filled with the oil of high refractive index (n) to increase the path of light.
$$\mathrm{d_{min}=\frac{1.22\lambda}{2n\:sin\beta}}$$
Such object lenses are called oil immersion objective lenses. The nsinβ term is called the numerical aperture NA.
$$\mathrm{d_{min}=\frac{1.22\lambda}{2(NA)}}$$
The Resolution of Microscope $\mathrm{R_M}$ is
$$\mathrm{R_M=\frac{1}{d_{min}}=\frac{2 (NA)}{1.22\lambda}=\frac{2\:nsin\beta}{1.22\lambda}}$$
Compound Microscope
In this microscope, light is used to illuminate the objects. The glass crystal lenses in it magnify the image of the object and make it fall on the retina of the observer's eye.
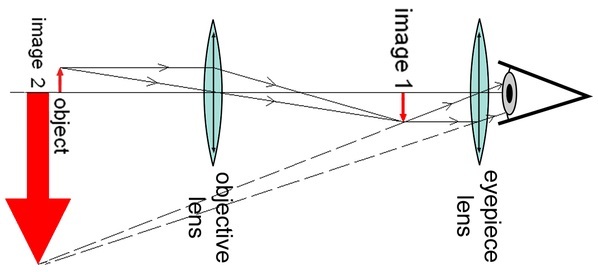
There are two lenses at the ends of the tube. The lens closest to the eye is called the eyepiece lens. The object is illuminated by light coming from below. This helps focus the light on the subject.
The lens that is closest to the object is called the objective lens. This lens creates a true, inverted and magnified image of the object. This image acts as the object for the second lens for the eyepiece.
An eyepiece acts like a simple microscope and produces a final magnified optical image.
When the inverted first image formed by the object lens is adjusted so that the eyepiece is close to the lens, but within its focal plane, the final image appears at a nearly infinite distance or near point.
The final image will be reversed to match the actual object.
Magnification of Compound Microscope
Fountains of Bryn Mawr, Microscope compound diagram, CC BY-SA 3.0
Ray Diagram of Compound Microscope
The lateral magnification of the object lens is as follows, (from the equation of lateral magnification of thin lenses)
$$\mathrm{m_0=\frac{h'}{h}}$$
$$\mathrm{tan\:β=\frac{h}{f_0}=\frac{h'}{L}}$$
$$\mathrm{\frac{h'}{h}=\frac{L}{f_0}}$$
$$\mathrm{m_0=\frac{L}{f_0}}$$
Here L is the distance between the first focal point of the eyepiece lens and the second focal point of the object lens. This is called the length (L) of the converging microscope tube and both $\mathrm{f_0}$ and $\mathrm{f_e}$ are less than L. If the final image is at the near point, the magnification of the eyepiece lens is
$$\mathrm{m_e=1+\frac{D}{f_e}}$$
The total magnification of near point focusing is as follows
$$\mathrm{m=m_0 m_e=(\frac{L}{f_0})(1+\frac{D}{f_e})}$$
If the final image is at an infinite distance (normal focusing), the magnification of the eyepiece lens is
$$\mathrm{m_e=\frac{D}{f_e}}$$
The total magnification from the normal focusing is as follows
$$\mathrm{m=m_0 m_e=(\frac{L}{f_0})(\frac{D}{f_e})}$$
Conclusion
The scientific study of microscopic objects is called microscopy. Compound microscopes contain at least two lenses. In 1590 Hans Janssen and his son Zacharias Janssen developed the first compound microscope in the Netherlands. A simple microscope is a magnifying lens with a short focal length to obtain a straight magnified optical image of an object. By looking at the object with a microscope, details about the object are obtained. A good microscope should not only magnify the object but also resolve two points on the object that are separated by a minimum distance. In a compound microscope, light is used to illuminate the objects.
FAQs
Q1. What is Scanning Tunnelling Microscope?
Ans.A Scanning Tunnelling Microscope (STM) is a microscope that can look very closely at the surface of an object. This means that one can see with greater resolution (resolving power). Through this, individual atoms can also be identified.
Q2. Explain Atomic or scanning force microscopy
Ans.
Scanning force microscopy (SFM) is a microscopic instrument that can accurately show even the layers of atoms on the surface of an object.
It can even show structures smaller than the nanometre scale with great precision.
Shine light onto an object, focus the light with lenses and magnify it thousands of times more sharply than common laboratory microscopes.
Q3. What are the applications of lenses?
Ans.
Magnifying glasses are made of convex lenses. Used in the process of microscope manufacturing.
The virtual image is captured by cameras using a convex lens. Convex lenses of appropriate ability can be used in hypermetropia. The image can be accurately focused on the retina using a pair of appropriately convex lenses.
Myopia defects can be solved by using a capable concave lens. The image of an appropriately capable concave lens will bring it back to the retina. Thus, this defect is corrected.
Concave lenses are used in flashlights, binoculars, telescopes and eyeglasses.
Q4. What is a Prism?
Ans. A solid material which is made of glass is called a prism. It is made up of three rectangular planes that are not parallel to each other. One of the three faces is made up of scars. This rough surface is called the base of the prism. The two other faces are called deviations because they are polished.
Q5. What is an achromatic lens?
Ans. An achromatic lens is a lens unit designed to be achromatic. Achromatic lenses are usually used to bring red and blue waves to the same focus. These are usually made by combining lenses of different chromatic resolving power. One is a double concave lens and the other is a biconvex lens.
Q6. Explain about Mirrors
The mirror is an optical device which is used for Reflection of the light. The ordinary mirrors in daily life are made of silver coated on the back side of the glass. Convex mirrors, concave mirrors and plane mirrors are the types of mirrors.

