数据结构
网络
关系数据库管理系统
操作系统
Java
MS Excel
iOS
HTML
CSS
Android
Python
C 编程
C++
C#
MongoDB
MySQL
Javascript
PHP
物理学
化学
生物学
数学
英语
经济学
心理学
社会学
服装设计
法律研究C++ 中的二叉搜索树节点删除
假设我们有一个二叉搜索树。我们将取一个键 k，并且我们必须从 BST 中删除给定的键 k，并返回更新后的 BST。因此，如果树类似于：
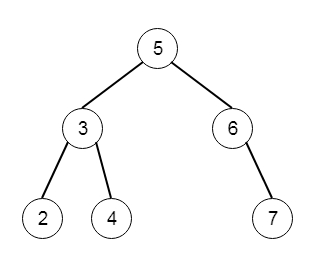
并且键 k = 3，则输出树将为：
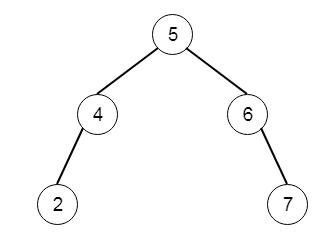
为了解决这个问题，我们将遵循以下步骤：
定义一个名为 deleteRoot() 的方法来删除根节点，这将按如下方式工作
如果根节点为空，则返回 null
如果根节点没有右子树，则返回根节点的左子树
x := 根节点的中序后继
将 x 的左子树设置为 left := 根节点的左子树
返回根节点的右子树
delete 方法将如下所示
如果根节点为空或根节点的值为键，则返回 deleteRoot(root)
curr := 根节点
创建一个无限循环，并执行以下操作
x := curr 节点的值
如果 key < x，则
如果 curr 的左子树为空或 curr 的左子树的值为 key，则
curr 的左子树 := deleteRoot(curr 的左子树) 并退出循环。
curr := curr 的左子树
否则
如果 curr 的右子树为空或 curr 的右子树的值为 key，则
curr 的右子树 := deleteRoot(curr 的右子树) 并退出循环。
curr := curr 的右子树
返回根节点
让我们看看以下实现以获得更好的理解：
示例
#include <bits/stdc++.h>
using namespace std;
class TreeNode{
public:
int val;
TreeNode *left, *right;
TreeNode(int data){
val = data;
left = NULL;
right = NULL;
}
};
void insert(TreeNode **root, int val){
queue<TreeNode*> q;
q.push(*root);
while(q.size()){
TreeNode *temp = q.front();
q.pop();
if(!temp->left){
if(val != NULL)
temp->left = new TreeNode(val);
else
temp->left = new TreeNode(0);
return;
} else {
q.push(temp->left);
}
if(!temp->right){
if(val != NULL)
temp->right = new TreeNode(val);
else
temp->right = new TreeNode(0);
return;
} else {
q.push(temp->right);
}
}
}
TreeNode *make_tree(vector<int> v){
TreeNode *root = new TreeNode(v[0]);
for(int i = 1; i<v.size(); i++){
insert(&root, v[i]);
}
return root;
}
void tree_level_trav(TreeNode*root){
if (root == NULL) return;
cout << "[";
queue<TreeNode *> q;
TreeNode *curr;
q.push(root);
q.push(NULL);
while (q.size() > 1) {
curr = q.front();
q.pop();
if (curr == NULL){
q.push(NULL);
}
else {
if(curr->left)
q.push(curr->left);
if(curr->right)
q.push(curr->right);
if(curr == NULL || curr->val == 0){
cout << "null" << ", ";
} else {
cout << curr->val << ", ";
}
}
}
cout << "]"<<endl;
}
class Solution {
public:
TreeNode* deleteNode(TreeNode* root, int key) {
if(root == NULL || root->val == key) return deleteRoot(root);
TreeNode* curr = root;
while(1) {
int x = curr->val;
if(key < x){
if(curr->left == NULL || curr->left->val == key){
curr->left = deleteRoot(curr->left);
break;
}
curr = curr->left;
} else {
if(curr->right == NULL || curr->right->val == key){
curr->right = deleteRoot(curr->right);
break;
}
curr = curr->right;
}
}
return root;
}
TreeNode* deleteRoot(TreeNode* root){
if(!root || root->val == 0)return NULL;
if(root->right == NULL) return root->left;
TreeNode* x = root->right;
while(x->left)x = x->left;
x->left = root->left;
return root->right;
}
};
main(){
vector<int> v = {5,3,6,2,4,NULL,7};
TreeNode *root = make_tree(v);
Solution ob;
tree_level_trav(ob.deleteNode(root, 3));
}输入
[5,3,6,2,4,null,7] 3
输出
[5, 4, 6, 2, null, 7, ]
更新于： 2020-04-30
4K+ 次查看
广告