数据结构
网络
RDBMS
操作系统
Java
MS Excel
iOS
HTML
CSS
Android
Python
C 编程
C++
C#
MongoDB
MySQL
Javascript
PHP
物理学
化学
生物学
数学
英语
经济学
心理学
社会学
时尚研究
法律研究在 C++ 中，如何将项目放置在 n^2 个位置，使得每行每列最多只有一个项目
在这个问题中，我们给定一个整数 n，表示垂直方向和水平方向各有 n 条线，这些线相交形成 n2 个交点。我们的任务是找到将 4 个项目放置在这些交点上的总方法数，
并且要求每行每列最多只能放置一个项目。
让我们举个例子来理解这个问题，
输入
n=4
输出
24
解释
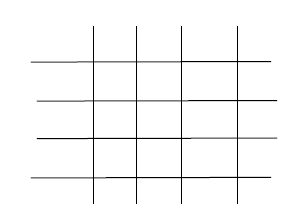
为了解决这个问题，我们需要从 n 条水平线中选择 4 条来放置项目，这可以通过 nC4 来计算。现在，每条水平线都有 n 条垂直线，因此在第一条选择的水平线上放置项目有 n 种方法。然后，我们移动到下一条选择的水平线，那里将有 n-1 种可能的放置方式。以此类推，第三个可以在 n-2 种方式中放置，第四个可以在 n-3 种方式中放置。因此，总方法数将是 nC4*n*(n-1)*(n-2)*(n-3)
程序展示算法的实现，
示例
#include <iostream>
using namespace std;
long long placeItems(int n) {
return (1LL * (1LL *
((n) * (n - 1) * (n - 2) * (n - 3)) / (4 * 3 * 2 * 1)) *
((1LL * (n) * (n - 1) * (n - 2) * (n - 3))));
}
int main() {
int n = 4;
cout<<"The number of way is which 4 items can be placed in the intersection of "<<n;
cout<<" lines vertically and horizotally are "<<placeItems(n);
return 0;
}输出
The number of way is which 4 items can be placed in the intersection of 4 lines vertically and horizotally are 24
更新于: 2020-07-17
63 次查看
广告