数据结构
网络
关系数据库管理系统(RDBMS)
操作系统
Java
MS Excel
iOS
HTML
CSS
Android
Python
C语言编程
C++
C#
MongoDB
MySQL
Javascript
PHP
物理学
化学
生物学
数学
英语
经济学
心理学
社会学
服装学
法学C++程序：检查有向图是否包含欧拉回路
欧拉回路/环路是一条路径；通过它我们可以恰好访问每条边一次。我们可以多次使用相同的顶点。欧拉环路是欧拉路径的一种特殊类型。当欧拉路径的起始顶点也与该路径的结束顶点相连时，则称其为欧拉环路。
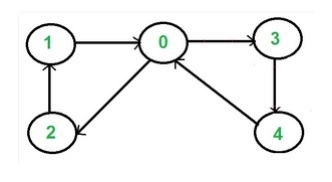
要检查图是否为欧拉图，我们必须检查两个条件：
图必须是连通的。
每个顶点的入度和出度必须相同。
输入 - 图的邻接矩阵。
| 0 | 1 | 0 | 0 | 0 |
| 0 | 0 | 1 | 0 | 0 |
| 0 | 0 | 0 | 1 | 1 |
| 1 | 0 | 0 | 0 | 0 |
| 0 | 0 | 1 | 0 | 0 |
输出 - 找到欧拉回路
算法
traverse(u, visited)
输入 - 起始节点u和已访问节点，用于标记已访问的节点。
输出 - 遍历所有连接的顶点。
Begin
mark u as visited
for all vertex v, if it is adjacent with u, do
if v is not visited, then
traverse(v, visited)
done
EndisConnected(graph)
输入 - 图。
输出 - 如果图是连通的则返回True。
Begin
define visited array
for all vertices u in the graph, do
make all nodes unvisited
traverse(u, visited)
if any unvisited node is still remaining, then
return false
done
return true
EndisEulerCircuit(Graph)
输入 - 给定的图。
输出 - 找到一个欧拉回路时返回True。
Begin if isConnected() is false, then return false define list for inward and outward edge count for each node for all vertex i in the graph, do sum := 0 for all vertex j which are connected with i, do inward edges for vertex i increased increase sum done number of outward of vertex i is sum done if inward list and outward list are same, then return true otherwise return false End
示例代码
#include<iostream>
#include<vector>
#define NODE 5
using namespace std;
int graph[NODE][NODE] = {{0, 1, 0, 0, 0},
{0, 0, 1, 0, 0},
{0, 0, 0, 1, 1},
{1, 0, 0, 0, 0},
{0, 0, 1, 0, 0}};
void traverse(int u, bool visited[]) {
visited[u] = true; //mark v as visited
for(int v = 0; v<NODE; v++) {
if(graph[u][v]) {
if(!visited[v])
traverse(v, visited);
}
}
}
bool isConnected() {
bool *vis = new bool[NODE];
//for all vertex u as start point, check whether all nodes are visible or not
for(int u; u < NODE; u++) {
for(int i = 0; i<NODE; i++)
vis[i] = false; //initialize as no node is visited
traverse(u, vis);
for(int i = 0; i<NODE; i++) {
if(!vis[i]) //if there is a node, not visited by traversal, graph is not connected
return false;
}
}
return true;
}
bool isEulerCircuit() {
if(isConnected() == false) { //when graph is not connected
return false;
}
vector<int> inward(NODE, 0), outward(NODE, 0);
for(int i = 0; i<NODE; i++) {
int sum = 0;
for(int j = 0; j<NODE; j++) {
if(graph[i][j]) {
inward[j]++; //increase inward edge for destination
vertex
sum++; //how many outward edge
}
}
outward[i] = sum;
}
if(inward == outward) //when number inward edges and outward edges
for each node is same
return true;
return false;
}
int main() {
if(isEulerCircuit())
cout << "Euler Circuit Found.";
else
cout << "There is no Euler Circuit.";
}输出
Euler Circuit Found.
更新于：2019年7月30日
495 次浏览
广告