数据结构
网络
RDBMS
操作系统
Java
MS Excel
iOS
HTML
CSS
Android
Python
C 编程
C++
C#
MongoDB
MySQL
Javascript
PHP
物理
化学
生物
数学
英语
经济学
心理学
社会研究
时装研究
法律研究一个 C++ 程序来检查对合矩阵
假设给定一个矩阵 M[r][c]，'r' 表示行数，'c' 表示列数，满足 r = c，形成一个方阵。我们需要检查给定的方阵是否为对合矩阵。
对合矩阵
当矩阵与自身相乘，结果是一个单位矩阵时，该矩阵被称为对合矩阵。单位矩阵 I 是一个沿主对角线元素均为 1，而其他元素均为 0 的矩阵。因此，我们可以说一个矩阵是对合矩阵当且仅当M*M=I，其中M 是某个矩阵，I 是单位矩阵。
如以下示例所示 −
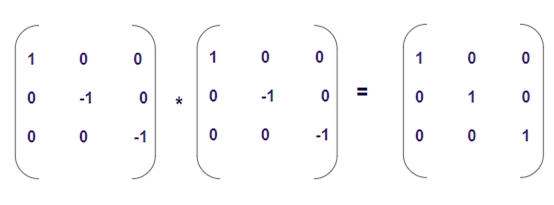
当我们把矩阵与自身相乘时，结果是单位矩阵；因此给定的矩阵是对合矩阵。
例
Input: { {1, 0, 0},
{0, -1, 0},
{0, 0, -1}}
Output: yes
Input: { {3, 0, 0},
{0, 2, 0},
{0, 0, 3} }
Output: no算法
Start
Step 1 -> define macro as #define size 3
Step 2 -> declare function for matrix multiplication.
void multiply(int arr[][size], int res[][size])
Loop For int i = 0 and i < size and i++
Loop For int j = 0 and j < size and j++
Set res[i][j] = 0
Loop For int k = 0 and k < size and k++
Set res[i][j] += arr[i][k] * arr[k][j]
End
End
End
Step 3 -> declare function to check involutory matrix or not
bool check(int arr[size][size])
declare int res[size][size]
Call multiply(arr, res)
Loop For int i = 0 and i < size and i++
Loop For int j = 0 and j < size and j++
IF (i == j && res[i][j] != 1)
return false
End
If (i != j && res[i][j] != 0)
return false
End
End
End
Return true
Step 4 -> In main()
Declare int arr[size][size] = { { 1, 0, 0 },
{ 0, -1, 0 },
{ 0, 0, -1 } }
If (check(arr))
Print its an involutory matrix
Else
Print its not an involutory matrix
Stop例
#include <bits/stdc++.h>
#define size 3
using namespace std;
// matrix multiplication.
void multiply(int arr[][size], int res[][size]){
for (int i = 0; i < size; i++){
for (int j = 0; j < size; j++){
res[i][j] = 0;
for (int k = 0; k < size; k++)
res[i][j] += arr[i][k] * arr[k][j];
}
}
}
// check involutory matrix or not.
bool check(int arr[size][size]){
int res[size][size];
multiply(arr, res);
for (int i = 0; i < size; i++){
for (int j = 0; j < size; j++){
if (i == j && res[i][j] != 1)
return false;
if (i != j && res[i][j] != 0)
return false;
}
}
return true;
}
int main(){
int arr[size][size] = { { 1, 0, 0 },
{ 0, -1, 0 },
{ 0, 0, -1 } };
if (check(arr))
cout << "its an involutory matrix";
else
cout << "its not an involutory matrix";
return 0;
}输出
its an involutory matrix
更新于： 2019 年 9 月 23 日
119 次浏览
广告