数据结构
网络
关系型数据库管理系统 (RDBMS)
操作系统
Java
MS Excel
iOS
HTML
CSS
Android
Python
C语言编程
C++
C#
MongoDB
MySQL
Javascript
PHP
物理学
化学
生物学
数学
英语
经济学
心理学
社会学
服装学
法学如果\( \sin \theta=\frac{3}{4} \)，证明\( \sqrt{\frac{\operatorname{cosec}^{2} \theta-\cot ^{2} \theta}{\sec ^{2} \theta-1}}=\frac{\sqrt{7}}{3} \)
已知
\( \sin \theta=\frac{3}{4} \)
要求
我们必须证明\( \sqrt{\frac{\operatorname{cosec}^{2} \theta-\cot ^{2} \theta}{\sec ^{2} \theta-1}}=\frac{\sqrt{7}}{3} \).
解：
设在直角三角形ABC中，∠B为直角，$\ sin\ \theta = sin\ A = \frac{3}{4}$。
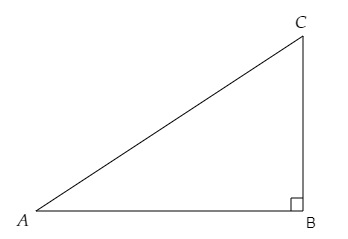
我们知道：
在以B为直角的直角三角形ABC中：
根据勾股定理：
$AC^2=AB^2+BC^2$
根据三角比的定义：
$sin\ \theta=\frac{对边}{斜边}=\frac{BC}{AC}$
$cosec\ \theta=\frac{斜边}{对边}=\frac{AC}{BC}$
$sec\ \theta=\frac{斜边}{邻边}=\frac{AC}{AB}$
$cot\ \theta=\frac{邻边}{对边}=\frac{AB}{BC}$
这里：
$AC^2=AB^2+BC^2$
$\Rightarrow (4)^2=AB^2+(3)^2$
$\Rightarrow AB^2=16-9$
$\Rightarrow AB=\sqrt{7}$
因此：
$cosec\ \theta=\frac{AC}{BC}=\frac{4}{3}$
$sec\ \theta=\frac{AC}{AB}=\frac{4}{\sqrt7}$
$cot\ \theta=\frac{AB}{BC}=\frac{\sqrt7}{3}$
现在：
让我们考虑左边：
$\sqrt{\frac{\operatorname{cosec}^{2} \theta-\cot ^{2} \theta}{\sec ^{2} \theta-1}}=\sqrt{\frac{\left(\frac{4}{3}\right)^{2} -\left(\frac{\sqrt{7}}{3}\right)^{2}}{\left(\frac{4}{\sqrt{7}}\right)^{2} -1}}$
$=\sqrt{\frac{\frac{16}{9} -\frac{7}{9}}{\frac{16}{7} -1}}$
$=\sqrt{\frac{\frac{16-7}{9}}{\frac{16-7}{7}}}$
$=\sqrt{\frac{\frac{9}{9}}{\frac{9}{7}}}$
$=\sqrt{1\times \frac{7}{9}}$
$=\sqrt{\frac{7}{9}}$
$=\frac{\sqrt{7}}{3}$
$=$ 右边
证毕。
90 次浏览
