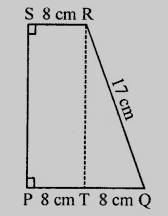
计算图形中梯形 PQRS 的面积。
 "\n
"\n
已知
梯形 PQRS
待做
我们必须求梯形 PQRS 的面积。
解答
在 △TQR 中,
∠RTQ=90o
这表示,
QR2=TQ2+RT2
(17)2=82+RT2
289=64+RT2
RT2=289−64
=225
=152
⇒RT=15 cm
PQ=8+8
=16 cm
梯形 PQRS 的面积= 12× 平行边和 × 高
=12(16+8)×15 cm2
=12×24×15
=180 cm2

广告
 "\n
"\n已知
梯形 PQRS
待做
我们必须求梯形 PQRS 的面积。
解答
在 △TQR 中,
∠RTQ=90o
这表示,
QR2=TQ2+RT2
(17)2=82+RT2
289=64+RT2
RT2=289−64
=225
=152
⇒RT=15 cm
PQ=8+8
=16 cm
梯形 PQRS 的面积= 12× 平行边和 × 高
=12(16+8)×15 cm2
=12×24×15
=180 cm2
