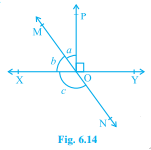
如图 6.14 所示，直线\( \mathrm{XY} \) 和 \( \mathrm{MN} \) 相交于 \( O \)。如果 \( \angle \mathrm{POY}=90^{\circ} \) 且 \( a: b=2: 3 \)，求 \( c \)。
"\n

已知
 直线 $XY, MN$ 相交于 $O$，$\angle POY=90^o$ 且 $a:b=2:3$。

 要求： 

我们要求出 $c$。

解

已知：

$\angle POY=90^o$ 且 $a:b=2:3$

我们知道：

线性对角的度数之和始终为 $180^o$。

这意味着：

$\angle POY+a+b=180^o$

将 $\angle POY=90^o$ 代入上式 

得到：

$90^o+a+b=180^o$

$a+b=180^o-90^o$

$a+b=90^o$

设 $a$ 为 $2x$，$b$ 为 $3x$（因为 $a:b=2:3$）

这意味着：

$2x+3x=90^o$

$5x=90^o$

$x=\frac{90^o}{5}$

$x=18^o$

因此，

$a=2\times18^o$

$a=36^o$ 且

$b=3\times18^o$

$b=54^o$

类似地，由于 $b$ 和 $c$ 也在一条直线上

得到：

$b+c=180^o$

这意味着：

$54^o+c=180^o$

$c=180^o-54^o$

$c=126^o$

因此，$c=126^o$。

教程点

更新于： 2022 年 10 月 10 日

38 次浏览

开启您的 职业生涯

通过完成课程获得认证

开始学习