在数轴上表示√3.5,√9.4和√10.5。
已知:
已知数为√3.5,√9.4和√10.5
要求:
我们需要在数轴上表示√3.5,√9.4和√10.5。
解:
1. 画一条线段AB=3.5单位长度。
2. 将B延长到点C,使得BC=1单位长度。
3. 找到AC的中点,设为O。
4. 以O为圆心,画一个经过A和C的半圆。
5. 画一条经过B且垂直于OB的直线,与半圆相交于D。
6. 以B为圆心,BD为半径画弧,交延长线OC于E。
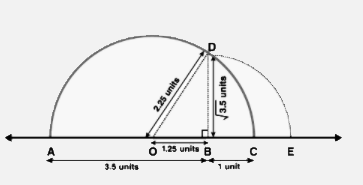
在直角三角形OBD中,
根据勾股定理,
BD2=OD2−OB2
=OC2−(OC−BC)2 [因为OD=OC]
BD2=2OC×BC−(BC)2
=2×2.25×1−1
=3.5
⇒BD=√3.5
1. 画一条线段AB=9.4单位长度。
按照上述步骤2到6进行操作。
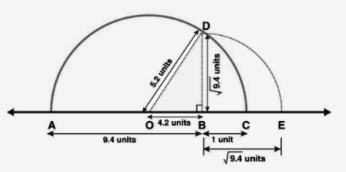
BD2=2OC×BC−(BC)2
=2×5.2×1−1
=9.4
⇒BD=√9.4
1. 画一条线段AB=10.5单位长度。
按照上述步骤2到6进行操作。
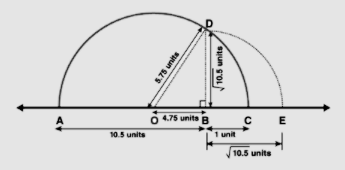
BD2=2OC×BC−(BC)2
=2×5.75×1−1
=10.5
⇒BD=√10.5

广告

 数据结构
数据结构 网络
网络 关系型数据库管理系统 (RDBMS)
关系型数据库管理系统 (RDBMS) 操作系统
操作系统 Java
Java iOS
iOS HTML
HTML CSS
CSS Android
Android Python
Python C语言编程
C语言编程 C++
C++ C#
C# MongoDB
MongoDB MySQL
MySQL Javascript
Javascript PHP
PHP