内接于等边三角形的圆的面积为$154\ cm^2$。求三角形的周长。[使用 $\pi = \frac{22}{7}$ 和 $\sqrt3= 1.73$]

已知

内接于等边三角形的圆的面积为$154\ cm^2$。

要求

我们必须求出三角形的周长。

解答

设半径为$r$的圆内接于等边三角形$ABC$。

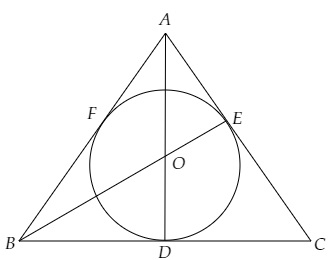
这意味着：

$OE=r$

$\Rightarrow \pi r^{2}=154$

$\Rightarrow \frac{22}{7} r^{2}=154$

$\Rightarrow r^{2}=\frac{154 \times 7}{22}$

$\Rightarrow r^{2}=49$

$\Rightarrow r^{2}=(7)^{2}$

$\Rightarrow r=7 \mathrm{~cm}$

因此：

$\mathrm{OE}=7 \mathrm{~cm}$

在等边三角形$\mathrm{ABC}$中，$AD\ \perp\ BC$，且$AD$平分$BC$于$D$。

$AD=3OD$

$=3 \times 7$

$=21 \mathrm{~cm}$ 设$a$为三角形$ABC$的边长。

$\Rightarrow \frac{\sqrt{3}}{2} a=21$

$\Rightarrow a=\frac{21 \times 2}{\sqrt{3}}$

$\Rightarrow a=\frac{21 \times 2 \times \sqrt{3}}{(\sqrt{3})^2}$

$\Rightarrow a=\frac{21 \times 2 \sqrt{3}}{3}$

$\Rightarrow a=7 \times 2 \sqrt{3}$

$\Rightarrow a=7 \times 2(1.73)$

$\Rightarrow a=24.22 \mathrm{~cm}$

三角形的周长 $=3a$

$=3 \times 24.22$

$=72.66 \mathrm{~cm}$

$=72.7 \mathrm{~cm}$

三角形的周长为$72.7 \mathrm{~cm}$。

教程点

更新于：2022年10月10日

188 次浏览

开启你的职业生涯

完成课程获得认证

开始学习