构建连续时间系统框图的基本元素

系统实现

连续时间系统的实现意味着获得与系统微分方程或传递函数相对应的网络。

框图

一个系统图，其中主要部分或功能由方块表示，方块之间由表示方块关系的线连接，称为该系统的框图。

Explore our latest online courses and learn new skills at your own pace. Enroll and become a certified expert to boost your career.

构建连续时间系统框图的元素

连续时间系统的传递函数可以通过使用积分器或微分器来实现。但是，由于某些缺点，微分器不用于实现实际系统。微分器的主要缺点是它们会放大高频噪声信号，而积分器则会抑制高频噪声信号。因此，为了实现实际的连续时间系统，仅使用积分器。除了积分器和微分器之外，加法器和乘法器也用于构建连续时间系统的框图。

因此，用于构建连续时间系统框图的基本元素为：

  • 积分器

  • 加法器

  • 乘法器

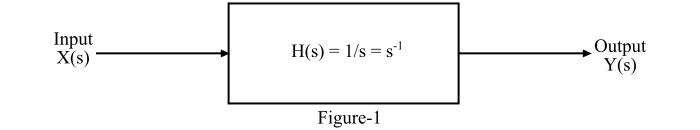
积分器

  • 积分器是用于对系统输入信号进行积分的元件。

  • 理想积分器的传递函数由下式给出：

H(s)=1s=s−1

  • 在连续时间系统实现中使用积分器的主要优点是积分器可以抑制高频噪声信号。由于这个原因，仅使用积分器来实现实际的连续时间系统。

  • 理想积分器的框图表示如图 1 所示。

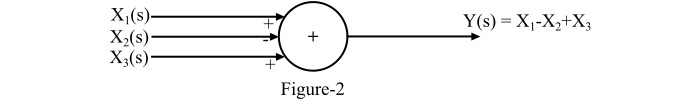
加法器

  • 加法器是用于执行信号加法和减法的元件。

  • 它也称为求和电路。

  • 加法器的输出是所有输入变量的代数和。

  • 在图形上，它由一个小圆圈表示，该圆圈至少有两个输入端和一个输出端，如图 2 所示。

乘法器

  • 乘法器是用于将输入信号乘以常数的元件。

  • 乘法器的增益（或常数）可以是正数或负数。

  • 乘法器增益的大小可以大于或小于 1。

  • 乘法器的图形表示如图 3 所示。

何时连续时间系统在物理上不可实现？

考虑由以下微分方程表示的nth阶连续时间系统：

dndtny(t)+an−1dn−1dtn−1y(t)+an−2dn−2dtn−2y(t)+...+a1ddty(t)+a0y(t)=bmdmdtmx(t)+bm−1dm−1dtm−1x(t)+bm−2dm−2dtm−2x(t)+...+b1ddtx(t)+b0x(t)

进行拉普拉斯变换，得到：

⇒sn+an−1sn−1+an−2sn−2+...+a1s+a0=bmsm+bm−1sm−1+bm−2sm−2+...+b1s+b0

因此，系统的传递函数为：

H(s)=Y(s)X(s)=bmsm+bm−1sm−1+bm−2sm−2+...+b1s+b0sn+an−1sn−1+an−2sn−2+...+a1s+a0

当 m > n 时，即传递函数中分子多项式的阶数大于分母多项式的阶数，则给定系统是非因果系统，因此在物理上不可实现。

更新于： 2022-01-24

824 次查看

开启您的 职业生涯

通过完成课程获得认证

开始学习
广告