单位冲激函数和单位阶跃函数的拉普拉斯变换

---

拉普拉斯变换

拉普拉斯变换是一种数学工具，用于将时域中的微分方程转换为频域或s域中的代数方程。

数学上，如果$\mathit{x}\mathrm{\left(\mathit{t}\right)}$是时域函数，则其拉普拉斯变换定义为：

$$\mathrm{\mathit{L}\mathrm{\left[ \mathit{x}\mathrm{\left(\mathit{t}\right)}\right]}\:\mathrm{=}\:\mathit{X}\mathrm{\left(\mathit{s}\right)}\:\mathrm{=}\:\int_{-\infty}^{\infty}\mathit{x}\mathrm{\left(\mathit{t}\right)}\mathit{e^{-st}}\:\mathit{dt}\:\:\:\:\:\:...(1)}$$

公式(1)给出了函数$\mathit{x}\mathrm{\left(\mathit{t}\right)}$的双边拉普拉斯变换。但对于因果信号，则应用单边拉普拉斯变换，其定义为：

$$\mathrm{\mathit{L}\mathrm{\left[ \mathit{x}\mathrm{\left(\mathit{t}\right)}\right]}\:\mathrm{=}\:\mathit{X}\mathrm{\left(\mathit{s}\right)}\:\mathrm{=}\:\int_{\mathrm{0}}^{\infty }\mathit{x}\mathrm{\left(\mathit{t}\right)}\mathit{e^{-st}}\:\mathit{dt}\:\:\:\:\:\:...(2)}$$

冲激函数的拉普拉斯变换

冲激函数定义为：

$$\mathrm{\mathit{\delta}\mathrm{\left(\mathit{t}\right)}\mathrm{=}\begin{cases} 1& \text{ 当 } t= 0 \ 0 & \text{ 当 } t \neq 0 \end{cases}}$$

因此，根据拉普拉斯变换的定义，我们有：

$$\mathrm{\mathit{X}\mathrm{\left(\mathit{s}\right)}\mathrm{=}\mathit{L\mathrm{\left[\mathit{\delta}\mathrm{\left(\mathit{t}\right)} \right]}}\:\mathrm{=}\:\int_{\mathrm{0}}^{\infty}\mathit{\delta}\mathrm{\left(\mathit{t}\right)}\mathit{e^{-st}}\:\mathit{dt}}$$

$$\mathrm{\Rightarrow \mathit{L\mathrm{\left[\mathit{\delta}\mathrm{\left(\mathit{t}\right)}\right]}}\:\mathrm{=}\:\mathrm{\left[\mathit{e^{-st}} \right]_{\mathit{t=\mathrm{0}}}}\:\mathrm{=}\:\mathrm{1}}$$

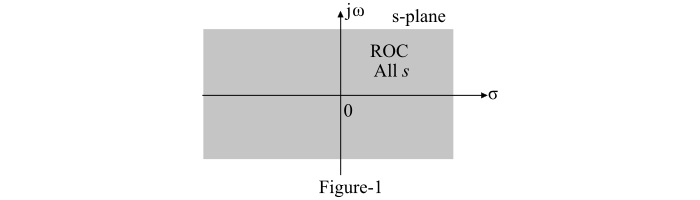
冲激函数拉普拉斯变换的收敛域(ROC)是整个s平面，如图1所示。因此，冲激函数的拉普拉斯变换及其ROC为：

$$\mathrm{\mathit{\delta}\mathrm{\left(\mathit{t}\right)}\overset{\mathit{LT}}{\leftrightarrow}\mathrm{1}\:\mathrm{且ROC\to 全部}\:\mathit{s}}$$

Explore our latest online courses and learn new skills at your own pace. Enroll and become a certified expert to boost your career.

阶跃函数的拉普拉斯变换

单位阶跃函数定义为：

$$\mathrm{\mathit{u}\mathrm{\left(\mathit{t}\right)}\mathrm{=}\begin{cases} 1& \text{ 当 } t\geq 0 \ 0 & \text{ 当 } t< 0 \end{cases}}$$

因此，根据拉普拉斯变换的定义，我们得到：

$$\mathrm{\mathit{X}\mathrm{\left(\mathit{s}\right)}\:\mathrm{=}\:\mathit{L\mathrm{\left[\mathit{u}\mathrm{\left(\mathit{t}\right)} \right]}}\:\mathrm{=}\:\int_{\mathrm{0}}^{\infty}\mathit{u}\mathrm{\left(\mathit{t}\right)}\mathit{e^{-st}}\:\mathit{dt}}$$

$$\mathrm{\Rightarrow \mathit{L\mathrm{\left[\mathit{u}\mathrm{\left(\mathit{t}\right)} \right]}}\:\mathrm{=}\:\int_{\mathrm{0}}^{\infty}\mathit{e^{-st}}\:\mathit{dt}\:\mathrm{=}\:\mathrm{\left[\frac{\mathit{e^{-st}}}{-\mathit{s}}\right]^{\infty}_{\mathrm{0}}}}$$

$$\mathrm{\Rightarrow \mathit{L\mathrm{\left[\mathit{u}\mathrm{\left(\mathit{t}\right)} \right]}}\:\mathrm{=}\:\mathrm{\left[\frac{\mathit{e^{-\infty}}-\mathit{e^{\mathrm{0}}}}{-\mathit{s}}\right]\:\mathrm{=}\:\frac{1}{\mathit{s}}}}$$

当$\mathit{Re}\mathrm{\left(\mathit{s} \right )}>\mathrm{0}$时，上述积分收敛，即单位阶跃函数的拉普拉斯变换的ROC为$\mathit{Re}\mathrm{\left(\mathit{s} \right )}>\mathrm{0}$，如图2所示。因此，阶跃函数的拉普拉斯变换及其ROC为：

$$\mathrm{\mathit{u}\mathrm{\left(\mathit{t}\right)}\overset{\mathit{LT}}{\leftrightarrow}\frac{1}{\mathit{s}}\:\mathrm{且ROC\to Re\mathrm{\left(\mathit{s}\right)}}>\mathrm{0}}$$