从一栋$7 \mathrm{~m}$高的建筑物顶部，测得一电缆塔顶部的仰角为$60^{\circ}$，测得其底部的俯角为$45^{\circ}$。确定塔的高度。

已知

从一栋$7 \mathrm{~m}$高的建筑物顶部，测得一电缆塔顶部的仰角为$60^{\circ}$，测得其底部的俯角为$45^{\circ}$。

要求

我们必须确定塔的高度。

解:  

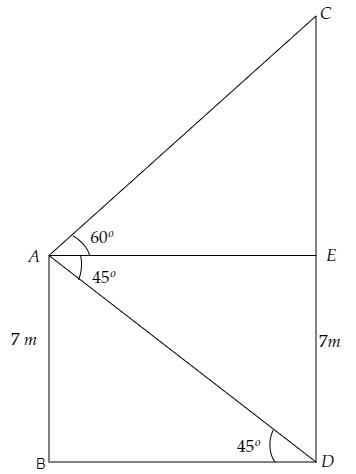
设$AB$为建筑物的高度，$CD$为电缆塔的高度。

设点$A$为观察点。

从图中，

$\mathrm{AB}=\mathrm{DE}=7 \mathrm{~m}, \angle \mathrm{ADB}=\angle \mathrm{EAD}=45^{\circ}, \angle \mathrm{CAE}=60^{\circ}$

设电缆塔的高度为$\mathrm{CD}=h \mathrm{~m}$，建筑物与塔之间的距离为$\mathrm{BD}=\mathrm{AE}=x \mathrm{~m}$。

这意味着，

$\mathrm{CE}=h-7 \mathrm{~m}$

我们知道，

$\tan \theta=\frac{\text { 对边 }}{\text { 邻边 }}$

$=\frac{\text { AB }}{BD}$

$\Rightarrow \tan 45^{\circ}=\frac{7}{x}$

$\Rightarrow 1=\frac{7}{x}$

$\Rightarrow x=7 \mathrm{~m}$.........(i)

同样地，

$\tan \theta=\frac{\text { 对边 }}{\text { 邻边 }}$

$=\frac{\text { CE }}{AE}$

$\Rightarrow \tan 60^{\circ}=\frac{h-7}{x}$

$\Rightarrow \sqrt3=\frac{h-7}{7}$              [来自 (i)]

$\Rightarrow 7\sqrt3=h-7 \mathrm{~m}$

$\Rightarrow h=7\sqrt3+7 \mathrm{~m}$            

$\Rightarrow h=7(\sqrt3+1) \mathrm{~m}$

因此，塔的高度为$7(\sqrt3+1) \mathrm{~m}$。

教程点

更新于: 2022年10月10日

87 次浏览

开启你的职业生涯

通过完成课程获得认证

开始学习