数据结构
网络
关系型数据库管理系统
操作系统
Java
MS Excel
iOS
HTML
CSS
Android
Python
C 语言编程
C++
C#
MongoDB
MySQL
Javascript
PHP
物理学
化学
生物学
数学
英语
经济学
心理学
社会学
时尚研究
法律研究从塔底仰望建筑物顶部的仰角为\( 30^{\circ} \)，从建筑物底仰望塔顶的仰角为\( 60^{\circ} \)。如果塔高\( 50 \mathrm{~m} \)，求建筑物的高度。
已知
从塔底仰望建筑物顶部的仰角为\( 30^{\circ} \)，从建筑物底仰望塔顶的仰角为\( 60^{\circ} \)。
塔高\( 50 \mathrm{~m} \)。
要求
我们需要求出建筑物的高度。
解:
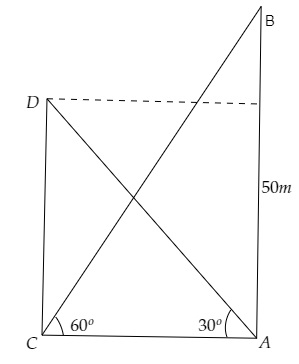
设塔的高度为$AB$，建筑物的高度为$CD$。
从图中可知，
$\mathrm{AB}=50 \mathrm{~m}, \angle \mathrm{BCA}=60^{\circ}, \angle \mathrm{DAC}=30^{\circ}$
设建筑物的高度为$\mathrm{CD}=h \mathrm{~m}$，建筑物和塔之间的距离为$\mathrm{CA}=x \mathrm{~m}$。
我们知道，
$\tan \theta=\frac{\text { 对边 }}{\text { 邻边 }}$
$=\frac{\text { AB }}{CA}$
$\Rightarrow \tan 60^{\circ}=\frac{50}{x}$
$\Rightarrow \sqrt3=\frac{50}{x}$
$\Rightarrow x=\frac{50}{\sqrt3} \mathrm{~m}$.........(i)
类似地，
$\tan \theta=\frac{\text { 对边 }}{\text { 邻边 }}$
$=\frac{\text { DC }}{AC}$
$\Rightarrow \tan 30^{\circ}=\frac{h}{x}$
$\Rightarrow \frac{1}{\sqrt3}=\frac{h}{\frac{50}{\sqrt3}}$ [根据 (i)]
$\Rightarrow \frac{1}{\sqrt3}\times\frac{50}{\sqrt3}=h \mathrm{~m}$
$\Rightarrow h=\frac{50}{3} \mathrm{~m}$
因此，建筑物的高度为$\frac{50}{3} \mathrm{~m}$。
134 次查看
