信号与系统 – 什么是四分之一波对称性？

四分之一波对称性

具有奇对称性或偶对称性以及半波对称性的周期函数$x(t)$被称为具有四分之一波对称性。

数学上，如果周期函数$x(t)$满足以下条件，则称其具有四分之一波对称性：

$$\mathrm{x(t)=x(-t)\:或\:x(t)=-x(-t)\:且\:x(t)=-x\left (t ± \frac{T}{2}\right )}$$

图1显示了一些具有四分之一波对称性的周期函数示例。

具有四分之一波对称性的函数的傅里叶级数系数计算如下：

情况一 – 当n为奇数时

$$\mathrm{x(t)=-x(-t)\:且\:x(t)=-x\left (t ± \frac{T}{2}\right )}$$

对于这种情况，

$$\mathrm{a_{0}=0\:\:且\:\:a_{n}=0}$$

并且，

$$\mathrm{b_{n}=\frac{8}{T} \int_{0}^{T/4}x(t)\:sin\:n\omega_{0}\:t\:dt}$$

情况二 – 当n为偶数时

$$\mathrm{x(t)=x(-t)\:且\:x(t)=-x\left (t ± \frac{T}{2}\right )}$$

对于这种情况，

$$\mathrm{a_{0}=0\:\:且\:\:b_{n}=0}$$

并且，

$$\mathrm{a_{n}=\frac{8}{T} \int_{0}^{T/4}x(t)\:cos\:n\omega_{0}\:t\:dt}$$

数值例子

使用四分之一波对称性，求图2所示波形的三角傅里叶级数。

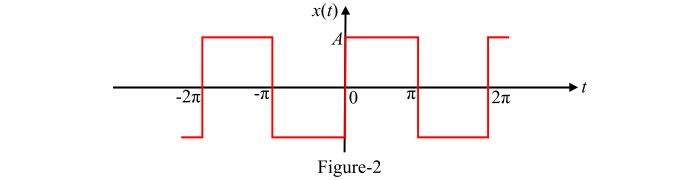
解答

从图2所示的波形可以看出，它具有奇对称性和半波对称性，因为它满足以下条件：

$$\mathrm{x(t)=-x(-t)\:且\:x(t)=-x\left (t ± \frac{T}{2}\right )}$$

因此，给定波形具有四分之一波对称性。因此，三角傅里叶级数系数为：

$$\mathrm{a_{0}=0\:\:且\:\:a_{n}=0}$$

系数$b_{n}$由下式给出：

$$\mathrm{b_{n}=\frac{8}{T} \int_{0}^{T/4}x(t)\:sin\:n\omega_{0}\:t\:dt}$$

此外，对于此函数，只有奇次谐波存在。因此，

• 波形的周期为：

$$\mathrm{T= 2\pi}$$

• 基频为：

$$\mathrm{\omega_{0}=\frac{2\pi}{T}=\frac{2\pi}{2\pi}=1}$$

$$\mathrm{\therefore\:b_{n}=\frac{8}{T} \int_{0}^{T/4}x(t)\:sin\:n\omega_{0}\:t\:dt=\frac{8}{2\pi}\int_{0}^{\pi/2}A\:sin\:nt\:dt}$$

$$\mathrm{\Rightarrow\:b_{n}=\frac{4A}{\pi}\left [ \frac{-cos\:nt}{n} \right ]_{0}^{\pi/2}=-\frac{4A}{n\pi}[cos(n\pi/2)-cos\:0]}$$

$$\mathrm{\therefore\:b_{n}=\begin{cases}\frac{4A}{n\pi} & 当n为奇数时\\0 & 当n为偶数时\end{cases}}$$

因此，波形的三角傅里叶级数为：

$$\mathrm{x(t)=\frac{4A}{\pi}sin\:t+\frac{4A}{3\pi}sin\:3t+\frac{4A}{5\pi}sin\:5t+....}$$

Saini Manish Kumar

更新于：2021年12月7日

4K+ 次浏览

启动您的职业生涯

完成课程获得认证

开始学习