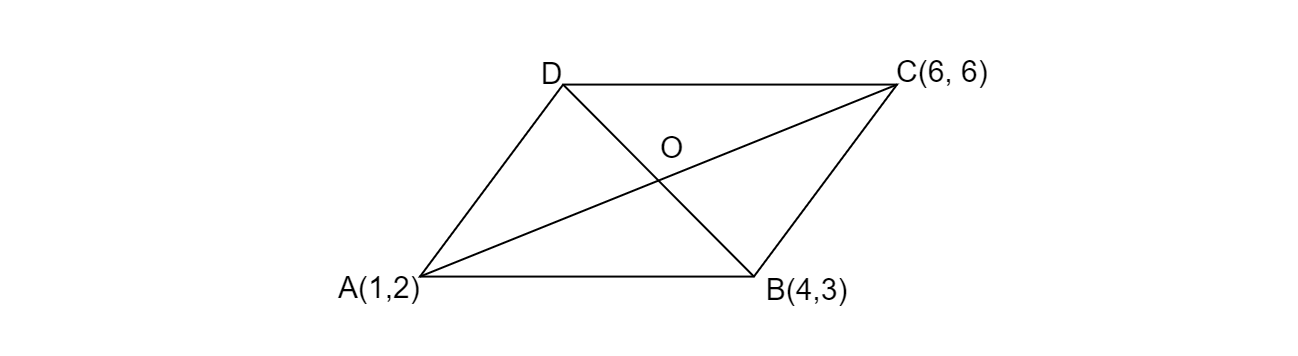
如果平行四边形ABCD的三个顶点为A(1,2)、B(4,3)和C(6,6)，求第四个顶点D的坐标。

已知：平行四边形ABCD，顶点为A(1, 2), B(4, 3) 和 C(6, 6)

求解：求第四个顶点D的坐标。

这里ABCD是一个平行四边形，AC和BD是互相平分的对角线，且相交于点O。

O是AC和BD的中点。

如果O是AC的中点。

$\Rightarrow O=\left(\frac{1+6}{2} ,\ \frac{2+6}{2}\right)$

$\therefore O=\left(\frac{7}{2} ,4\right)$

O也是BD的中点。

设D点坐标为(x, y)。

$\therefore O=\left(\frac{( x+4)}{2} ,\frac{( y+3)}{2}\right)$

但我们知道O$=\left(\frac{7}{2} ,4\right)$

$\therefore \left(\frac{7}{2} ,4\right) =\left(\frac{( x+4)}{2} ,\frac{( y+3)}{2}\right)$

$\Rightarrow \ \ \frac{( x+4)}{2} =\frac{7}{2} \ and\ \frac{( y+3)}{2} =4$

$\Rightarrow x+4=7$ and $y+3=8$

$\Rightarrow$

$x=7-4=3$ and $y=8-3=5$

因此 $D=( 3,\ 5)$

第四个顶点D的坐标为(3, 5)