从山顶上，观察到正东方向的两块相邻的千米路标的俯角分别为 $30^{\circ}$ 和 $45^{\circ}$。求这两块路标到山脚的距离。

已知

从山顶上，观察到两个连续千米路标的俯角为

45°和 30°

求：

这两块路标到山脚的距离。

解

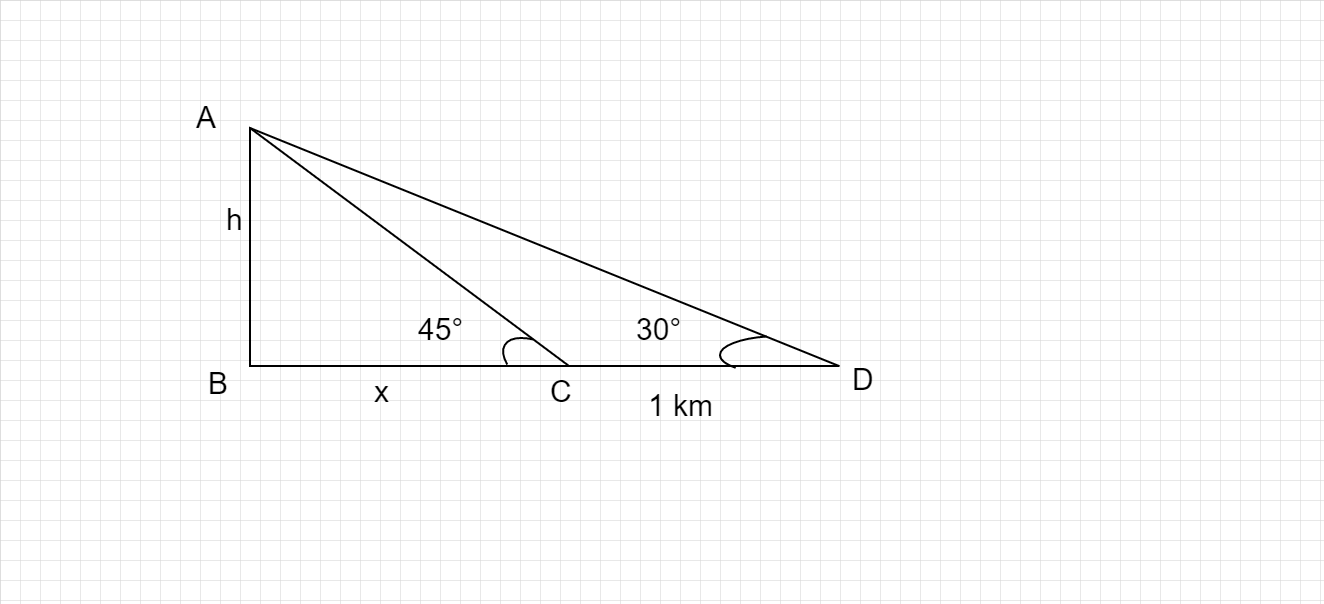
山高 AB = h

距离 BC = x km

距离 CD = 1 km

在 ABC 中                                                                                               $$\displaystyle tan\ \theta \ =\ \frac{对边}{邻边} \ $$

$$\displaystyle tan\ 45=\ \frac{h}{x} \ $$                                                         (tan 45° = 1)

   $$\displaystyle 1\ =\ \frac{h}{x}$$

h = x .....................................................(i)

在 ABD 中

$$\displaystyle tan\ 30\ =\ \frac{h}{x\ +\ 1} \ $$

$$\displaystyle \frac{1}{\sqrt{3}} \ =\ \frac{h}{x\ +\ 1} \ $$

交叉相乘，

$$\displaystyle x\ +\ 1\ =\ \sqrt{3} h$$  

改写，

$$\displaystyle \ \sqrt{3} h\ =\ x\ +\ 1\ ...........................................( ii)$$

将 (i) 代入 (ii)

$$\displaystyle \ \sqrt{3} \ x\ =\ x\ +\ 1\ $$

$$\displaystyle \ \sqrt{3} \ x\ -\ x\ =\ 1\ $$

提取 x 为公因数，

$$\displaystyle x\ \left(\sqrt{3} \ -\ 1\right) \ =\ 1$$

$$\displaystyle x\ =\ \frac{1}{\sqrt{3} \ -\ 1}$$

取共轭，

$$\displaystyle x\ =\ \frac{1}{\sqrt{3} \ -\ 1} \ \times \frac{\sqrt{3} \ +\ 1}{\sqrt{3} \ +\ 1}$$

$$\displaystyle x\ =\ \frac{\sqrt{3} \ +\ 1}{\left(\sqrt{3} \ \ \right)^{2} -\ ( 1)^{2}}$$

$$\displaystyle x\ =\ \frac{\sqrt{3} \ +\ 1}{3\ -\ 1}$$

$$\displaystyle x\ =\ \frac{\sqrt{3} \ +\ 1}{2}$$

√3 = 1.732

$$\displaystyle x\ =\ \frac{\ 1.732+\ 1}{2}$$

$$\displaystyle x\ =\ \frac{\ 2.732}{2}$$

x = 1.366

距离 BC = 1.366 km

第一个路标到山脚的距离 = 1.366 km

第二个路标到山脚的距离

$$\displaystyle BD\ =\ BC\ +\ CD$$

$$\displaystyle BD\ =\ 1.366\ +\ 1$$

距离 = 2.366 km

第二个路标到山脚的距离 = 2.366 km

教程点

更新于: 2022年10月10日

99 次查看

开启你的职业生涯

完成课程，获得认证

开始学习