从以下四个选项中选择正确答案
如果\( \triangle \mathrm{ABC} \sim \Delta \mathrm{QRP}, \frac{\operatorname{ar}(\mathrm{ABC})}{\operatorname{ar}(\mathrm{PQR})}=\frac{9}{4}, \mathrm{AB}=18 \mathrm{~cm} \)且\( \mathrm{BC}=15 \mathrm{~cm} \)，则\( \mathrm{PR} \)等于
(A) \( 10 \mathrm{~cm} \)
(B) \( 12 \mathrm{~cm} \)
(C) \( \frac{20}{3} \mathrm{~cm} \)
(D) \( 8 \mathrm{~cm} \)
已知
\( \triangle \mathrm{ABC} \sim \Delta \mathrm{QRP}, \frac{\operatorname{ar}(\mathrm{ABC})}{\operatorname{ar}(\mathrm{PQR})}=\frac{9}{4}, \mathrm{AB}=18 \mathrm{~cm} \)且\( \mathrm{BC}=15 \mathrm{~cm} \).
要求
我们必须找到\( \mathrm{PR} \)的度量。
解答
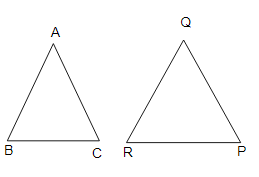
我们知道，
两个相似三角形的面积之比等于它们对应边平方之比。
因此，
$\frac{\operatorname{ar}(\triangle A B C)}{\operatorname{ar}(\Delta Q R P)}=\frac{(B C)^{2}}{(R P)^{2}}$
$\frac{\operatorname{ar}(\triangle A B C)}{\operatorname{ar}(\triangle P Q R)}=\frac{9}{4}$ (已知)
这意味着，
$\frac{(15)^{2}}{(R P)^{2}}=\frac{9}{4}$
$(R P)^{2}=\frac{225 \times 4}{9}$
$(PR)^2=100$
$PR=10 \mathrm{~cm}$
- 相关文章
- \( \triangle \mathrm{ABC} \sim \triangle \mathrm{PQR} . \quad \) 如果 \( \quad \mathrm{AB}+\mathrm{BC}=12 \mathrm{~cm} \) \( \mathrm{PQ}+\mathrm{QR}=15 \mathrm{~cm} \)且\( \mathrm{AC}=8 \mathrm{~cm} \)，求\( \mathrm{PR} \)。
- 如果\( \Delta \mathrm{ABC} \sim \Delta \mathrm{DEF}, \mathrm{AB}=4 \mathrm{~cm}, \mathrm{DE}=6 \mathrm{~cm}, \mathrm{EF}=9 \mathrm{~cm} \)且\( \mathrm{FD}=12 \mathrm{~cm} \)，求\( \triangle \mathrm{ABC} \)的周长。
- 从以下四个选项中选择正确答案：如果在两个三角形\( \mathrm{ABC} \)和\( \mathrm{PQR} \)中，\( \frac{\mathrm{AB}}{\mathrm{QR}}=\frac{\mathrm{BC}}{\mathrm{PR}}=\frac{\mathrm{CA}}{\mathrm{PQ}} \)，则(A) \( \triangle \mathrm{PQR} \sim \triangle \mathrm{CAB} \)(B) \( \triangle \mathrm{PQR} \sim \triangle \mathrm{ABC} \)(C) \( \triangle \mathrm{CBA} \sim \triangle \mathrm{PQR} \)(D) \( \triangle \mathrm{BCA} \sim \triangle \mathrm{PQR} \)
- \( \triangle \mathrm{ABC} \sim \triangle \mathrm{ZYX} . \) 如果\( \mathrm{AB}=3 \mathrm{~cm}, \quad \mathrm{BC}=5 \mathrm{~cm} \)，\( \mathrm{CA}=6 \mathrm{~cm} \)且\( \mathrm{XY}=6 \mathrm{~cm} \)，求\( \Delta \mathrm{XYZ} \)的周长。
- 从以下四个选项中选择正确答案：已知\( \triangle \mathrm{ABC} \sim \triangle \mathrm{DFE}, \angle \mathrm{A}=30^{\circ}, \angle \mathrm{C}=50^{\circ}, \mathrm{AB}=5 \mathrm{~cm}, \mathrm{AC}=8 \mathrm{~cm} \)且\( D F=7.5 \mathrm{~cm} \)。则以下说法正确：(A) \( \mathrm{DE}=12 \mathrm{~cm}, \angle \mathrm{F}=50^{\circ} \)(B) \( \mathrm{DE}=12 \mathrm{~cm}, \angle \mathrm{F}=100^{\circ} \)(C) \( \mathrm{EF}=12 \mathrm{~cm}, \angle \mathrm{D}=100^{\circ} \)(D) \( \mathrm{EF}=12 \mathrm{~cm}, \angle \mathrm{D}=30^{\circ} \)
- \( \Delta \mathrm{ABC} \sim \Delta \mathrm{XZY} \)。如果\( \triangle \mathrm{ABC} \)的周长为\( 45 \mathrm{~cm} \)，\( \triangle \mathrm{XYZ} \)的周长为\( 30 \mathrm{~cm} \)且\( \mathrm{AB}=21 \mathrm{~cm} \)，求\( \mathrm{XY} \)。
- 在\( \triangle \mathrm{ABC} \)中，\( \mathrm{AD} \)是中线。如果\( \mathrm{AB}=18 \mathrm{~cm} \)。\( \mathrm{AC}=14 \mathrm{~cm} \)且\( \mathrm{AD}=14 \mathrm{~cm} \)，求\( \triangle \mathrm{ABC} \)的周长。
- 如图，\( \mathrm{ABC} \)和\( \mathrm{BDE} \)是两个等边三角形，使得\( \mathrm{D} \)是\( \mathrm{BC} \)的中点。如果\( \mathrm{AE} \)与\( \mathrm{BC} \)相交于\( \mathrm{F} \)，证明(i) \( \operatorname{ar}(\mathrm{BDE})=\frac{1}{4} \operatorname{ar}(\mathrm{ABC}) \)(ii) \( \operatorname{ar}(\mathrm{BDE})=\frac{1}{2} \operatorname{ar}(\mathrm{BAE}) \)(iii) \( \operatorname{ar}(\mathrm{ABC})=2 \) ar \( (\mathrm{BEC}) \)(iv) \( \operatorname{ar}(\mathrm{BFE})=\operatorname{ar}(\mathrm{AFD}) \)(v) \( \operatorname{ar}(\mathrm{BFE})=2 \operatorname{ar}(\mathrm{FED}) \)(vi) \( \operatorname{ar}(\mathrm{FED})=\frac{1}{8} \operatorname{ar}(\mathrm{AFC}) \)[提示：连接\( \mathrm{EC} \)和\( \mathrm{AD} \)。证明\( \mathrm{BE} \| \mathrm{AC} \)和\( \mathrm{DE} \| \mathrm{AB} \)
- 已知\( \triangle \mathrm{ABC} \sim \Delta \mathrm{EDF} \)，使得\( \mathrm{AB}=5 \mathrm{~cm} \)，\( \mathrm{AC}=7 \mathrm{~cm} \)，\( \mathrm{DF}=15 \mathrm{~cm} \)且\( \mathrm{DE}=12 \mathrm{~cm} \)。求三角形其余边的长度。
- \( \mathrm{D}, \mathrm{E} \)和\( \mathrm{F} \)分别是\( \triangle \mathrm{ABC} \)的边\( \mathrm{BC}, \mathrm{CA} \)和\( \mathrm{AB} \)的中点。证明(i) BDEF 是平行四边形。(ii) \( \operatorname{ar}(\mathrm{DEF})=\frac{1}{4} \operatorname{ar}(\mathrm{ABC}) \)(iii) \( \operatorname{ar}(\mathrm{BDEF})=\frac{1}{2} \operatorname{ar}(\mathrm{ABC}) \)
- 作一个三角形\( \mathrm{ABC} \)，其中\( \mathrm{BC}=8 \mathrm{~cm} \)，\( \angle \mathrm{B}=45^{\circ} \)且\( \mathrm{AB}-\mathrm{AC}=3.5 \mathrm{~cm} \)。
- 求四边形\( \mathrm{ABCD} \)的面积，其中\( \mathrm{AB}=3 \mathrm{~cm} \)，\( \mathrm{BC}=4 \mathrm{~cm} \)，\( \mathrm{CD}=4 \mathrm{~cm} \)，\( \mathrm{DA}=5 \mathrm{~cm} \)且\( \mathrm{AC}=5 \mathrm{~cm} \)。
- 作一个三角形\( \mathrm{ABC} \)，其中\( \mathrm{BC}=7 \mathrm{~cm} \)，\( \angle \mathrm{B}=75^{\circ} \)且\( \mathrm{AB}+\mathrm{AC}=13 \mathrm{~cm} \)。
- 如图，如果\( \angle \mathrm{A}=\angle \mathrm{C} \)，\( \mathrm{AB}=6 \mathrm{~cm} \)，\( \mathrm{BP}=15 \mathrm{~cm} \)，\( \mathrm{AP}=12 \mathrm{~cm} \)且\( \mathrm{CP}=4 \mathrm{~cm} \)，则求\( \mathrm{PD} \)和CD的长度。"
- 从以下四个选项中选择正确答案：已知\( \triangle \mathrm{ABC} \sim \triangle \mathrm{PQR} \)，且\( \frac{\mathrm{BC}}{\mathrm{QR}}=\frac{1}{3} \)。则\( \frac{\text { ar (PRQ) }}{\operatorname{ar}(\mathrm{BCA})} \)等于(A) 9(B) 3(C) \( \frac{1}{3} \)(D) \( \frac{1}{9} \)
数据结构
网络
关系型数据库管理系统
操作系统
Java
MS Excel
iOS
HTML
CSS
Android
Python
C语言编程
C++
C#
MongoDB
MySQL
Javascript
PHP
物理
化学
生物
数学
英语
经济学
心理学
社会学
时尚研究
法律研究