数据结构
网络
关系型数据库管理系统
操作系统
Java
MS Excel
iOS
HTML
CSS
Android
Python
C 语言编程
C++
C#
MongoDB
MySQL
Javascript
PHP
物理
化学
生物
数学
英语
经济学
心理学
社会学
时尚研究
法律研究从一座 100 米高的灯塔顶端观察海平面，两艘船的俯角分别为 $30^{o}$ 和 $45^{o}$。如果一艘船正好在另一艘船的后面，并且都在灯塔的同一侧，求这两艘船之间的距离。[使用 $\sqrt{3}=1.732$]
已知：灯塔的高度 $=100\ m$，两艘船的俯角分别为 $30^{o}$ 和 $45^{o}$，并且一艘船正好在另一艘船的后面，都在灯塔的同一侧。
要求：求这两艘船之间的距离。
解答
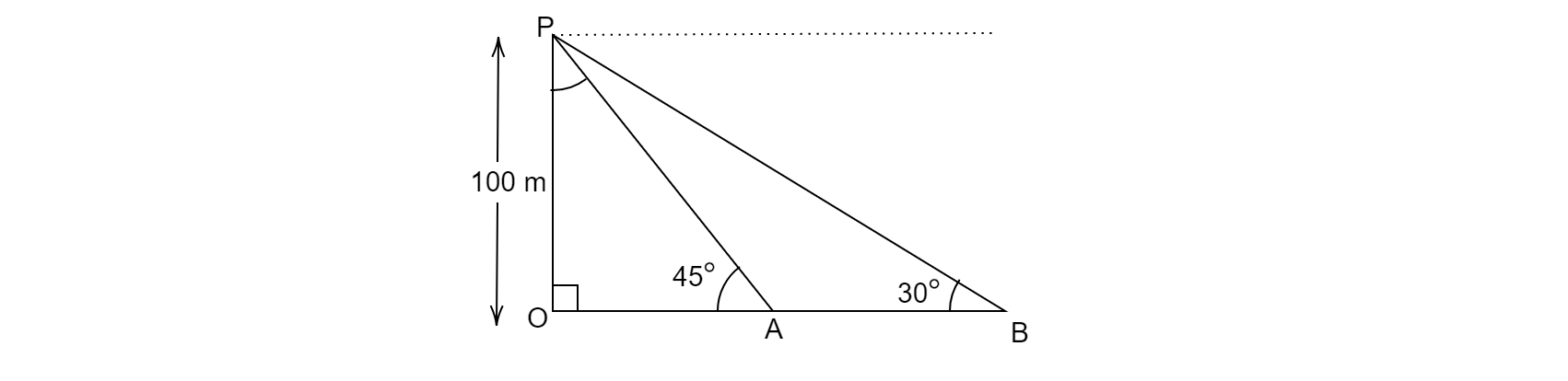
设两艘船之间的距离为 $x$
在 $\vartriangle APO$ 中
$tan45^{o}=\frac{100}{y}=1$
$\Rightarrow y=100\ m$ ...$( i)$
在 $\vartriangle POB$ 中，
$tan30^{o}=\frac{OP}{OB}=\frac{100}{x+y}=\frac{1}{\sqrt{3}}$
$\Rightarrow x+y=100\sqrt{3} $
将 $( i)$ 中 $y=100\ m$ 代入，
$x+100=100\sqrt{3}$
$\Rightarrow x=100(\sqrt{3}-1)$
$\Rightarrow x=100(1.732-1)$
$\Rightarrow x=73.2\ m$
$\therefore$ 两艘船相距 $73.2\ 米$。
更新于： 2022年10月10日
236 次浏览
广告