能力倾向 - 高度与距离在线测验

以下测验提供与高度与距离相关的多项选择题 (MCQ)。您需要阅读所有给出的答案，然后点击正确的答案。如果您不确定答案，可以使用显示答案按钮查看答案。您可以使用下一题按钮查看测验中的新一组问题。

问题 1 - 一个站在垂直观测塔顶端的人观察到一辆以匀速行驶的汽车正朝塔驶来。如果俯角从 45° 变为 60° 需要 10 分钟，那么汽车在此之后多久到达观测塔？

答案：B

解释

Height & Distance Solution 3

Let AB be the tower and C and D be the two positions of the car.
Then,from figure
AB/AC=tan 60 =√3 => AB=√3AC
AB/AD=tan 45=1 => AB=AD
AB=AC+CD
CD=AB-AC=√3AC - AC=AC (√3-1)
CD = AC (√3-1) =>10 min
 AC=> ?
AC/(AC(√3-1)) x 10=?=10/(√3-1)=13.66=13 min 20 sec(approx)

问题 2 - 当太阳高度角从 45° 变为 60° 时，一座塔的影子长度减少了 45 米。这座塔的高度是多少？

答案：A

解释

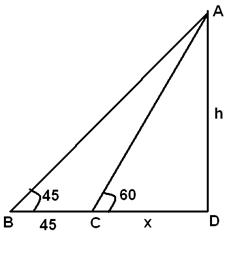
Height & Distance Solution 6

Let AD be the tower, BD be the initial shadow and CD be the final shadow.
Given that BC = 45 m,  ABD = 45°,  ACD = 60°,
Let CD = x, AD = h
From the right   CDA, tan60=h/x
From the right   BDA, tan45=(45+x)/h=>h=45+x
=>h=45+h/√3
=>h(1-1/√3)=45
=>h=45/(1-1/√3)=(45√3)/(√3-1)

问题 3 - 一棵直立的树因雷雨而折断。断裂的部分弯曲，使得树尖触地，形成一个仰角 45°。树尖到树根的距离是 20 米。那么这棵树的高度是多少？

答案：A

解释

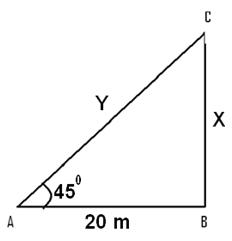
Height & Distance Solution 9

Let the total length of the tree be X+Y meters
From the figure tan 45=X/20 =>X=20
cos 45 = 20/Y =>Y=20/cos 45 =20√2
X+Y=20+20radic;2=20+2x10x1.414 =48.28 meters

问题 4 - 一根 10 米高的旗杆立在一座 50 米高的建筑物上。一个观察者在 60 米的高度上，观察旗杆和建筑物顶端所成的角相等。观察者到旗杆顶端的距离是多少？

答案：C

解释

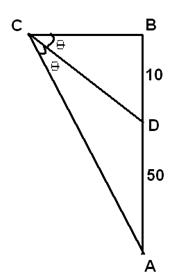
Height & Distance Solution 10

From the figure
tanθ=10/CB
tan(2θ)=60/CB=(2tan(θ))/(1-tan(θ)²)
=>60/CB=(2tan(θ))/(1-tan(θ)²)=(2(10/CB))/(1-(10/CB)²)
=>3/1=1/(1-(10/CB)²)
=>3x(1-(10/CB)sup>2)=1
3CB²-300=CB²
2CB²=300=>CB=√150=5√6

问题 5 - 考虑一个高 6 米的垂直竖井，其影子长 2√3 米，求太阳的仰角？

答案：A

解释

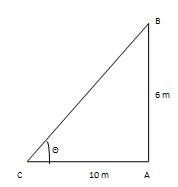
Height & Distance Solution 13

Let AB be the building and AC be its shadow. Then, AB= 6m and AC= 2√3m.
Let ∠ACB= θ Then tan θ = AB/AC= 6/2√3m
 =√3= >θ =60°
Point of rise of the sun is 60°

问题 6 - 从距离塔底 50 米处测得塔顶的仰角为 30°。塔的高度是多少？

答案：B

解释

Height & Distance Solution 16

Let AB be the tower and AC be the even line such that AC=50 m and ∠ACB=30°.
AB/AC=tan 30°=1/√3
=>x/50 = 1/√3
> x=50*1/√3m= 50/√3m.
∴ Height of the tower=50/√3m.

问题 7 - 从一个高 90 米的悬崖顶端，观察到一座塔的顶部和底部的俯角分别为 30° 和 60°。这座塔的高度是多少？

答案：C

解释

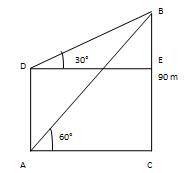
Height & Distance Solution 21

Let AB be the precipice and CD be the tower. Draw DE || CA.
Then, ∠BDE=30°, ∠BCA=60°and AB= 90m.
From right △CAB, we have
CA/AB=cost60°=1/√3 => CA/90=1/√3
=>CA=(90*1/√3* √3/√3)
=30 √3m.
∴ DE =CE=30/√3m.
From right ?DEB, we have
BE/DE= tan30°=1/√3 => BE/30 √3=1 √3
=>BE= (30 √3*1 √3) =30m.
∴ CD=AE= (AB-BE) = (90-30) m=60m.
Hence, the tower's stature is 60m.

问题 8 - 在水平地面上，有一座竖直的塔，塔顶有一根旗杆。在距离塔 9 米处，观察到旗杆顶端和底部的仰角分别为 60° 和 30°。旗杆的高度是多少？

答案：A

解释

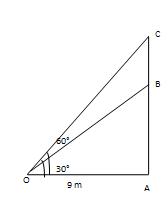
Height & Distance Solution 25

Let AB be the tower and BC be the flag pole and let O be the point of observation.
Then, A=9m, ∠AOB=30°and ∠AOC=60°
AB/OA=tan30°=1 ∠ =>AB/9=1∠
=>AB=(9*1/ √3* √3/√3)= 3 √3m.
AC/AO=tan60°=√3 =>AC/9= √3 =>AC= 9√3m.
∴BC= (AC-AB) = (9 √3-3 √3) m=6 √3m.
∴ Height of the flagpole is 6 √m.

aptitude_height_distance.htm

打印页面